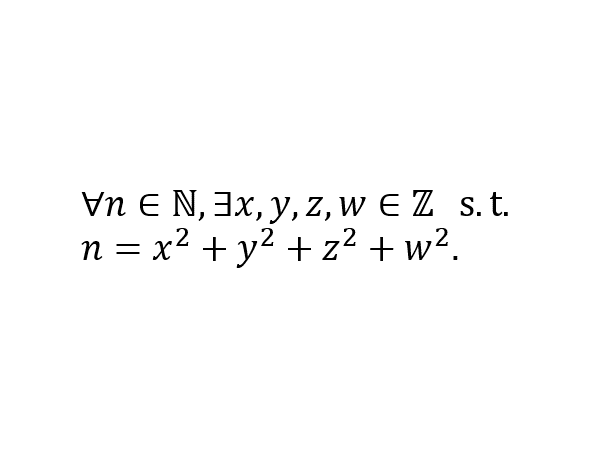Casa
four square theorem

Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project

Por um escritor misterioso

Descrição
Any natural number can be represented as the sum of the squares of four non-negative integers. For most numbers there are multiple representations. In this Demonstration the four integers (not squared) may be viewed using three different options.1. For each set of four numbers a polygon is displayed with vertices and .2. Each set is sorted from smallest to largest. The first two numbers a;;
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Moments-based interface reconstruction, remap and advection - ScienceDirect
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Prime Numbers
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Lagrange's four-square theorem
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Visual Computing Institute - RWTH Aachen University
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
How do we know that the optimal solution of a Linear Programming Problem lies at one of the vertices? - Quora
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
He wrote, in Communications of the ACM: I believe
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
SOLVED: Theorem 8.35 (Lagrange's Four-Square Theorem) then n can be expressed as the sum If n is a number; of four squares: natural lattice A in 4-space is a set of the
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Alan Schoen geometry
de por adulto (o preço varia de acordo com o tamanho do grupo)