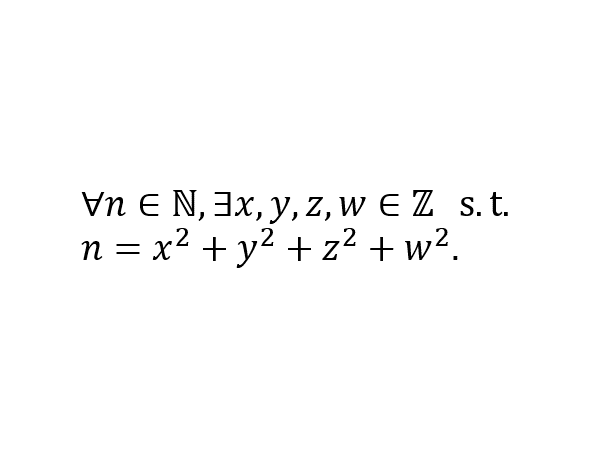PDF) Quarternions and the Four Square Theorem
Por um escritor misterioso
Descrição
The Four Square Theorem was proved by Lagrange in 1770: ev- ery positive integer is the sum of at most four squares of positive integers, i.e. n = A2 +B2 +C2 +D2;A;B;C;D 2 Z An interesting proof is presented here based on Hurwitz integers, a subset

Page:Scientific Papers of Josiah Willard Gibbs - Volume 2.djvu/130 - Wikisource, the free online library

PDF] New parametrization of $A^2+B^2+C^2=3D^2$ and Lagrange's four-square theorem
Modern algebra, Algebraic Structures, Rings & Group Theory

Quaternion algebras over global fields
The Pythagorean Theorem

Fast construction of accurate quaternion splines Proceedings of the 24th annual conference on Computer graphics and interactive techniques

PDF) Quarternions and the Four Square Theorem
Quaternions. “And here there dawned on me the notion…, by Priyam

Lagrange's four-square theorem - Wikipedia

Full article: Efficient data encryption technique using quaternions for wireless sensor network
On Quaternions and Octonions, by John Conway and Derek Smith

Minimal non-abelian nodal braiding in ideal metamaterials

PDF) Factorization of Hurwitz quaternions

The (Imaginary) Numbers at the Edge of Reality

PDF) A Simple Proof of Jacobi's Four-Square Theorem